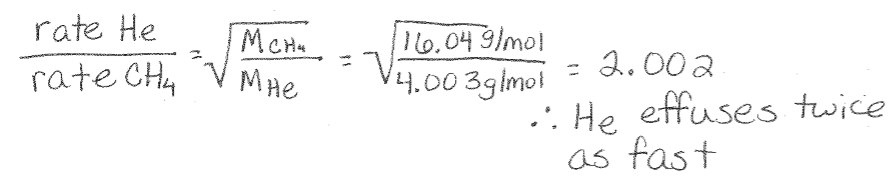The absolute temperature of a gas is a measure of the average kinetic energy. Gases in the same container have the same temperature, therefore they have the same average kinetic energy. However, some gas particles in the container are heavier and slower and some particles in the container are lighter and faster. (ie. There is a distribution of gas particles moving at different speeds related to their molar mass.) As temperature increases, the average kinetic energy of the gas molecules and their velocity increases. The speed of a gas molecule having average kinetic energy is called the root mean square (rms) speed and is abbreviated u.

RMS Speed Example: Calculate the rms speed of an O2 molecule at 31°C
Answer= 490m/s
Two vocabulary words often apply to the movement of gas particles: diffusion and effusion. Diffusion is the spread of gases from an area of high concentration to an area of low concentration. (Ex. A bottle of perfume is opened. The odor is strongest close to the bottle but gradually the scent diffuses throughout the room.) Effusion is the escape of gas molecules through a tiny hole. (Ex. A tire has a small hole in it from a nail. The tire will go flat because all gas particles will make their way through the opening.)
The rate of effusion and diffusion can be quantified using Graham’s law. Rate of gas movement is inversely proportional to the square root of its molar mass We can use the following equation to compare relative rms speed of two gases:

Graham’s Law Example: What is the rate of velocity of He-4 atoms compared to Ra- 222 when both gases are at the same temperature?

Graham’s Law Example: He and CH4 are placed in an effusion apparatus. Calculate the ratio of their effusion rates.